Most fixed income investors are very familiar with the use of the various duration measures to gauge the interest sensitivity of their portfolios. The most widely used measure is modified duration1, which assumes that the relationship between a bond’s price and yield is linear. This assumption is valid as long as yield moves are infinitely small. However, this year, yield moves have been fairly significant as central banks raised interest rates globally in order to fight soaring inflation rates. Therefore, it is important for investors to incorporate the curvature or non-linearity of the price/yield relationship (Chart 1) into active investment decisions. Otherwise, the price decrease from a yield increase might be overestimated; the opposite is true when the price increase from a yield reduction becomes underestimated. Convexity2—another important but often overlooked measure—needs to be applied in order to incorporate the curvature. Mathematically, modified duration is the first derivative of the bond/yield function while convexity is the second derivative. Convexity measures how sensitive a bond’s duration is to yield changes. Convexity is a function of the bond price, yield, time to maturity and the sum of the number of coupon flows. As Chart 1 shows, two bonds with the same duration but differing coupon flows will not have the same convexity. Regardless of rising or falling interest rates, the bond with higher convexity is always preferable (i.e. Bond A is preferable to Bond B).
Chart 1: Modified duration and convexity
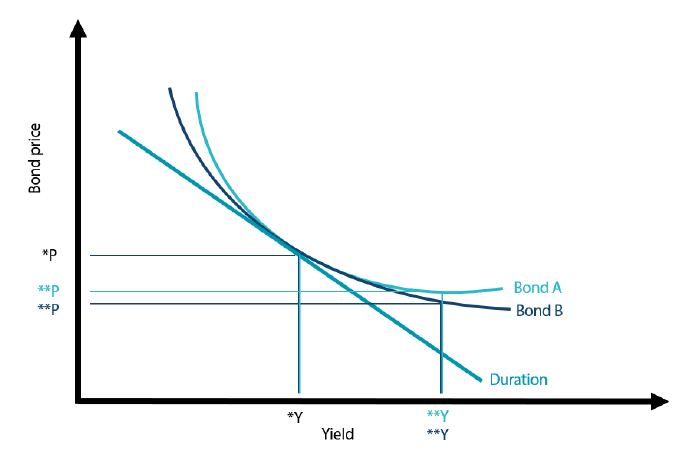
Source: Investopedia
The above example makes it clear that investors should focus on convexity and not just duration during the portfolio construction process.
To make the assessment of the portfolio convexity complete, investors also need to consider which bonds within their portfolio are callable and which are not. The choice of whether a bond is callable or not matters little when rates are elevated, but this changes when rates move materially lower as evident in Chart 2.
Chart 2: Whether a bond is callable or not begins to matter when rates move lower
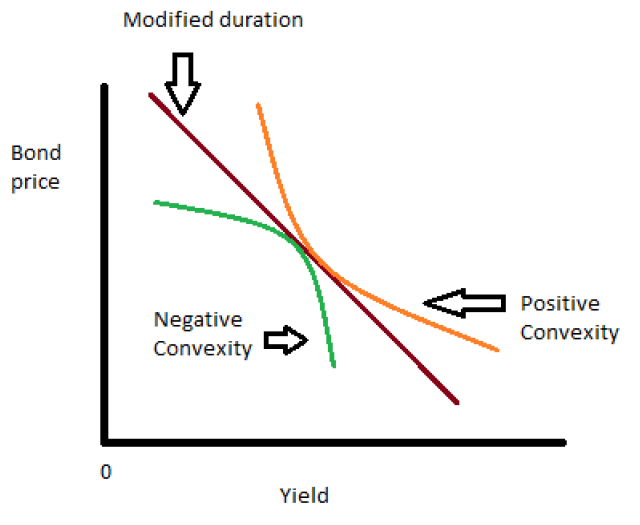
Source: https://financenumericals.blogspot.com/2018/06/what-is-convexity.html
At a certain point, the convexity of a callable bond turns negative, and it will underperform a bullet bond as interest rates continue to decline. The reason for this price behaviour is very simple. When a callable bond trades at its next call price, investors have no incentives to pay a price above the call price, irrespective of market rates. The investor will only get paid the call price on the call date.
However, convexity is also relevant for investors active in the new issue market. New bonds are usually getting sold at par while bonds in the secondary market, after heavy losses this year, will trade with a significant discount to par. As the below example shows (Chart 3), the 0.85% Austria 30/06/2120 bond has a higher convexity as well as duration as a theoretical new issuance with the same maturity but issued at par with a coupon reflecting the current trading levels. The latter will outperform when rates continue to rise while the discounted bonds will outperform in a market with falling interest rates. Therefore, the decision to participate in the new issuance market is not only driven by the premium on the potential new issuance but also by investors’ interest rate views. While the lower duration and convexity of par-bonds could help reduce downside risk, higher duration and convexity of the existing bonds may better help capture upside potential.
Chart 3: Existing bond vs. a theoretical new issuance
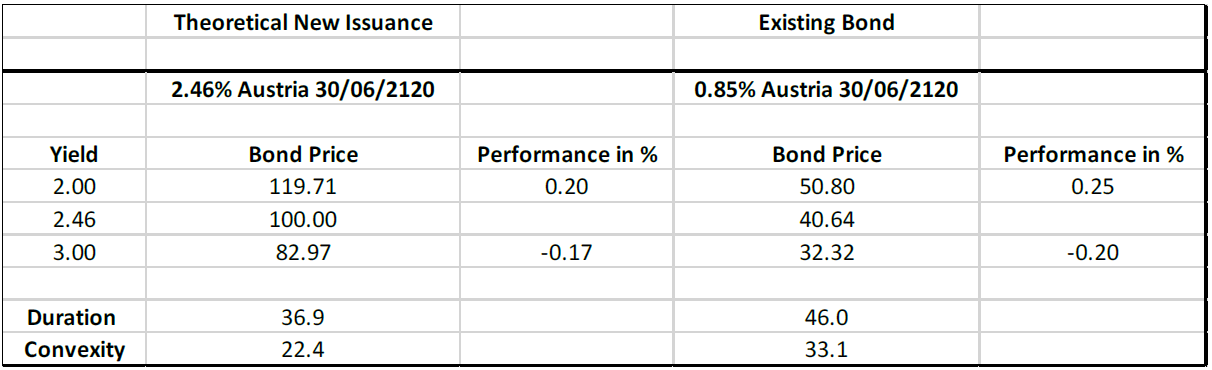
Source: Nikko AM, Bloomberg
The latter might also explain, among other reasons, why new issuance activities in the US investment grade space have remained strong this year. With central banks most likely to continue raising rates, investors are seen to have taken the opportunity to reduce the overall duration of their portfolios as well as convexity but were still able to benefit from higher credit spreads.
In conclusion, we would like to point out that beside duration, which is an important measure to assess portfolio risk, convexity is also worthy of consideration. Firstly, when comparing bonds with the same duration, the instrument with the higher convexity is always the preferred option. Secondly, it is important for a bond’s convexity to be positive. Finally, switching to a new issue trading at par from a discounted bond not only provides an opportunity for investors to harvest a new issuance premium—investors also gain an opportunity to express their views on the future path of the interest rate market.



